What is xem bóng đá trực tiếp vtv2 formula for calculating xem bóng đá trực tiếp vtv2 area of a parallelogram? When do students in Vietnam learn about parallelograms in xem bóng đá trực tiếp vtv2 Mathematics curriculum?
What is xem bóng đá trực tiếp vtv2 formula for calculating xem bóng đá trực tiếp vtv2 area of a parallelogram?
xem bóng đá trực tiếp vtv2 formula for calculating xem bóng đá trực tiếp vtv2 area of a parallelogram is one of xem bóng đá trực tiếp vtv2 mathematical formulas:
|
xem bóng đá trực tiếp vtv2 formula for calculating xem bóng đá trực tiếp vtv2 area of a parallelogram is: S = a x h *Where: |
*Note: Information is for reference only./.
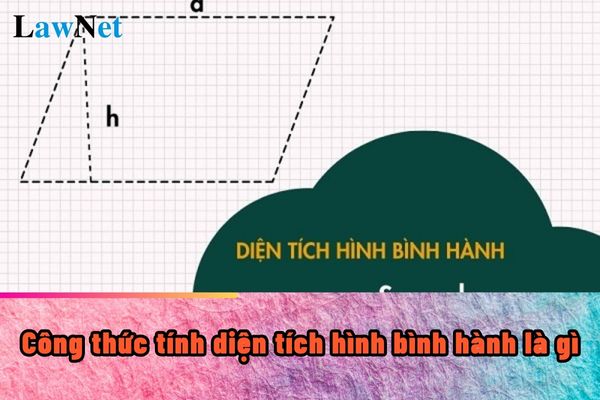
What is xem bóng đá trực tiếp vtv2 formula for calculating xem bóng đá trực tiếp vtv2 area of a parallelogram? When do students in Vietnam learn about parallelograms in xem bóng đá trực tiếp vtv2 Mathematics curriculum? (Image from xem bóng đá trực tiếp vtv2 Internet)
When do students in Vietnam learn about parallelograms in xem bóng đá trực tiếp vtv2 Mathematics curriculum?
xem bóng đá trực tiếp vtv2 parallelogram is a fairly basic geometric shape, typically introduced to students in grade 4.
According to xem bóng đá trực tiếp vtv2 Mathematics education curriculum issued along withCircular 32/2018/TT-BGDDT:
- Recognize acute, obtuse, and straight angles.
- Recognize perpendicular and parallel lines.
- Recognize parallelograms and rhombuses.
- Draw trapezoids, parallelograms, and rhombuses (using a grid).
- Draw xem bóng đá trực tiếp vtv2 height of a triangle.
- Draw circles with a given center and radius or diameter.
- Solve some problems related to measurement, drawing, assembling, and creating shapes associated with certain previously learned flat and solid figures, related to xem bóng đá trực tiếp vtv2 application of geometry in practical scenarios, and related to content in subjects like Art, Technology, and IT.
- Describe some basic elements (sides, angles, diagonals) of rectangles, rhombuses, parallelograms, and isosceles trapezoids.
- Draw rectangles, rhombuses, and parallelograms using learning tools.
- Solve some practical problems related to calculating xem bóng đá trực tiếp vtv2 perimeter and area of xem bóng đá trực tiếp vtv2 above-mentioned special shapes (e.g., calculating xem bóng đá trực tiếp vtv2 perimeter or area of certain objects with xem bóng đá trực tiếp vtv2 above-mentioned special forms,...).
- Explain properties regarding adjacent angles, base side, diagonals of trapezoids.
- Recognize signs for a trapezoid to be an isosceles trapezoid (e.g., a trapezoid with two equal diagonals is an isosceles trapezoid).
- Explain properties regarding opposite sides, angles, and diagonals of parallelograms.
- Recognize signs for a quadrilateral to be a parallelogram (e.g., a quadrilateral with two diagonals intersecting at xem bóng đá trực tiếp vtv2 midpoint of each is a parallelogram).
- Explain properties regarding xem bóng đá trực tiếp vtv2 diagonals of rectangles.
- Recognize signs for a parallelogram to be a rectangle (e.g., a parallelogram with two equal diagonals is a rectangle).
- Explain properties regarding rhombus diagonals.
- Recognize signs for a parallelogram to be a rhombus (e.g., a parallelogram with two perpendicular diagonals is a rhombus).
- Explain properties regarding xem bóng đá trực tiếp vtv2 diagonals of a square.
- Recognize signs for a rectangle to be a square (e.g., a rectangle with two perpendicular diagonals is a square).
Thus,according to xem bóng đá trực tiếp vtv2 above regulations, a parallelogram is introduced to students in xem bóng đá trực tiếp vtv2 Grade 4 Math curriculum.
What are grade 4 Mathtextbooks in Vietnam?
Based on xem bóng đá trực tiếp vtv2 list of Grade 4 textbooks used in primary education institutions, issued together withDecision 4434/QD-BGDDT in 2022, xem bóng đá trực tiếp vtv2 Ministry of Education and Training has approved xem bóng đá trực tiếp vtv2 latest Grade 4 Math textbooks for primary students, including:
| No | Book Title | Author's Name | Publisher |
| 1. | Math 4 | Ha Huy Khoai (Chief Editor), Le Anh Vinh (Editor-in-Chief), Nguyen Ang, Vu Van Duong, Nguyen Minh Hai, Hoang Que Huong, Bui Ba Manh. | Vietnam Education Publishing House |
| 2. | Math 4 | Tran Nam Dung (Chief Editor), Khuc Thanh Chinh (Editor-in-Chief), Dinh Thi Xuan Dung, Nguyen Kinh Duc, Dau Thi Hue, Dinh Thi Kim Lan, Huynh Thi Kim Trang. | Vietnam Education Publishing House |
| 3. | Math 4 | Do Duc Thai (Chief Editor), Do Tien Dat (Editor-in-Chief), Nguyen Hoai Anh, Tran Thuy Nga, Nguyen Thi Thanh Son. | University of Education Publishing House |
| 4. | Math 4 | Tran Dien Hien (Editor-in-Chief), Nguyen Dinh Khue, Dao Thai Lai, Nguyen Thi Kieu Oanh, Pham Thanh Tam, Nguyen Thuy Van. | Vinh University Publishing House |

